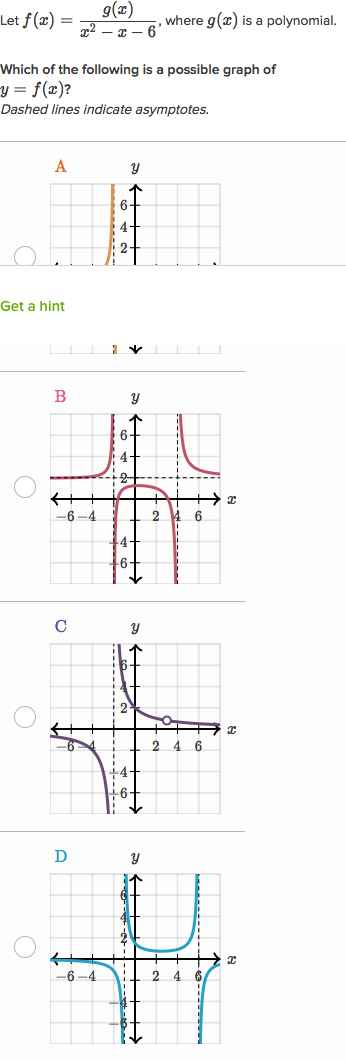
Rational Functions
Finding Horizontal Asymptotes of Rational Functions Remember that an asymptote is a line that the graph of a function approaches but never touches. Rational functions contain asymptotes, as seen in this example: In this example, there is a vertical asymptote at x = 3 and a horizontal asymptote at y = 1. The curves approach these asymptotes but never cross them. The method used to find the horizontal asymptote changes depending on how the degrees of the polynomials in the numerator and denominator of the function compare. If both polynomials are the same degree, divide the coefficients of the highest degree terms.
Example: Both polynomials are 2 nd degree, so the asymptote is at. If the polynomial in the numerator is a lower degree than the denominator, the x-axis (y = 0) is the horizontal asymptote. If the polynomial in the numerator is a higher degree than the denominator, there is no horizontal asymptote. There is a slant asymptote, which we will study in a later lesson. Examples: Find the horizontal asymptote of each rational function.
. Show Remarks/Examples. Graph functions expressed symbolically and show key features of the graph, by hand in simple cases and using technology for more complicated cases.
Graph linear and quadratic functions and show intercepts, maxima, and minima. Graph square root, cube root, and piecewise-defined functions, including step functions and absolute value functions. Graph polynomial functions, identifying zeros when suitable factorizations are available, and showing end behavior. Graph rational functions, identifying zeros and asymptotes when suitable factorizations are available, and showing end behavior. Graph exponential and logarithmic functions, showing intercepts and end behavior, and trigonometric functions, showing period, midline, and amplitude, and using phase shift. TASK RUBRIC Getting Started Misconception/Error The student is unable to produce an accurate representation of the graph.
Examples of Student Work at this Level The student is unable to correctly graph the rational function using technology because the student:. Does not understand how to use the graphing technology available.
Does not place parentheses around the denominator and graphs. The student correctly graphs the function with technology but:. Does not understand the graph (e.g., the distinction between the asymptote that is shown and the actual graph) and makes errors transferring it to paper. Questions Eliciting Thinking What type of function is this? How is this function different from a linear function or a quadratic function?
What are some of the features of the graph of a rational function? How would you enter this function into a graphing calculator? Is there a horizontal asymptote? Is there a vertical asymptote? How did you set the window on your calculator? Do you think you could see the most critical parts of the graph? Instructional Implications Provide the student with basic instruction on graphing with technology.

Review how to use the GRAPH and TABLE functions on the graphing calculator. Provide guidance on how to set the window. Provide the student with instruction on graphing rational functions including how to find asymptotes and intercepts. Review that the y-intercept of a function can be found by letting x = 0 and solving for y. Remind the student that finding the asymptotes are critical to graphing a rational function. Review the concept of an asymptote and the relationship between an asymptote and the graph of a rational function. Explain that vertical asymptotes are associated with values of x for which the function is undefined and show the student how to find vertical asymptotes.
To find the horizontal asymptote, remind the student to look at the degree in the numerator and the denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is y = 0. If the degree of the numerator is greater than the degree of the denominator, there is no horizontal asymptote. If the degree of the numerator is the same as the degree of the denominator, the horizontal asymptote is where a is the coefficient of the term with the highest degree in the numerator and b is the coefficient of the term with the highest degree in the denominator. Provide additional opportunities for the student to graph simple rational functions e.g.by hand and other rational functions e.g.using technology. Ask the student to describe the asymptotes (by giving their equations) and the intercepts (by giving their coordinates).
Have the student explore rational functions with Illustrative Mathematics’ interactive activity, Graphing Rational Functions. Moving Forward Misconception/Error The student is able to sketch the graph of the function but cannot describe the key features of the graph. Examples of Student Work at this Level The student correctly sketches the graph but:.
Does not understand the meaning of intercepts or how to describe them. Does not understand what happens to f(x) as x gets larger. Questions Eliciting Thinking Can you identify the points where the graph crosses the x-axis? What is a horizontal asymptote? Does this function have a horizontal asymptote?
What is the equation of this asymptote? What is a vertical asymptote? Does this function have a vertical asymptote? What is the equation of this asymptote? Look at your table of values.
What happens to y as x gets larger? What happens on the left side of the vertical asymptote? What happens at the vertical asymptote? What happens to the right of the vertical asymptote? Instructional Implications Review how to find the intercepts of a function and ask the student to find the y-intercept. Then ask the student to attempt to find the x-intercept. Explain that the result of this attempt means the function is undefined at f( x) = 0 which indicates there is a horizontal asymptote at f( x) = 0.
Review the concept of an asymptote and the relationship between an asymptote and the graph of a rational function. Explain that vertical asymptotes are associated with values of x for which the function is undefined and show the student how to find vertical asymptotes. To find the horizontal asymptote, remind the student to look at the degree in the numerator and the denominator. If the degree of the numerator is less than the degree of the denominator, the horizontal asymptote is y = 0. If the degree of the numerator is greater than the degree of the denominator, there is no horizontal asymptote. If the degree of the numerator is the same as the degree of the denominator, the horizontal asymptote is where a is the coefficient of the term with the highest degree in the numerator and b is the coefficient of the term with the highest degree in the denominator. Have the student explore rational functions with Illustrative Mathematics’ interactive activity, Graphing Rational Functions.
Almost There Misconception/Error The student provides an essentially correct response but uses imprecise language. Examples of Student Work at this Level The student correctly sketches the graph of the function but does not answer all questions completely. For example, the student:. Identifies or as an intercept but does not specify which intercept it is. Identifies the horizontal asymptote as 2. Identifies the vertical asymptote as 0.
Describes the values of f( x) as simply getting smaller as x gets larger and larger. Questions Eliciting Thinking You wrote that the intercept is. Which intercept is this?
Rational Functions Practice
What is the equation of the horizontal asymptote? What is the equation of the vertical asymptote? Can you be more specific about what happens to the values of f( x) as x gets larger and larger? How small will f( x) become? Will it ever be a negative value? Instructional Implications Provide feedback to the student regarding any error and allow the student to revise his or her work. Provide additional opportunities for the student to graph rational functions and describe features of their graphs.
Got It Misconception/Error The student provides complete and correct responses to all components of the task. Examples of Student Work at this Level The student correctly sketches the graph of the function as: And:. Identifies the y-intercept as and states there is no x-intercept. Identifies the horizontal asymptote as the line y = 0 or the x-axis, and the vertical asymptote as the line x = 2. States that as the value of x gets larger, the value of f( x) approaches, but never reaches, zero. Questions Eliciting Thinking Can you explain how you found the asymptotes?
Rational Functions
What is the domain of this function? What is the range of this function? Instructional Implications Challenge the student with more difficult rational functions to graph by hand and then allow the student to use technology to check his or her graph. Consider implementing other MFAS graphing tasks such as Graphing Root Functions (F-IF.3.7), Graphing an Exponential Function (F-IF.3.7), or Graphing a Step Function (F-IF.3.7).